Volume of a cone

A cone is a solid obtained by joining all rays originating from a single point (the apex of the cone) and passing directly through the flat surface. A circular cone is obtained by rotating a right triangle around one of its legs. For this reason, a circular cone is called a rotational cone.

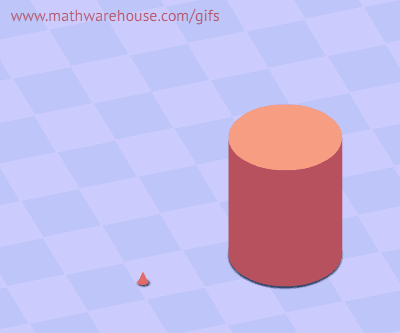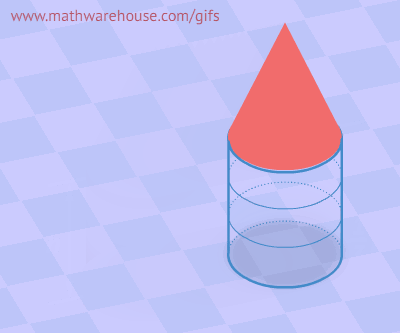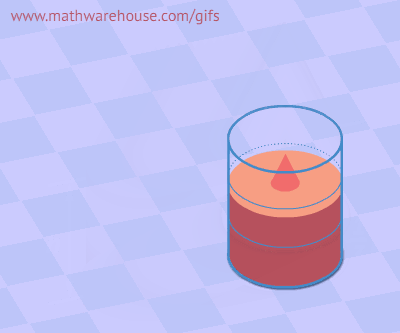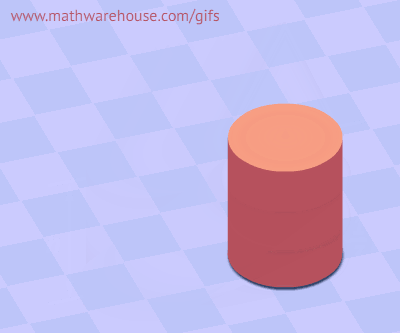
This triangle, to form the cone, must rotate around one of its legs, which is not only the axis of rotation but also the height of the cone. The other leg becomes the radius of the resulting circular base of the cone, and the hypotenuse is the slant height (height dropped at a right angle to the circle line, not the center).

Technically, the relationship of a cone with a cylinder is identical to the relationship of a pyramid with a cube (parallelepiped), the only difference is that the formula derivation goes through the ratios of their spherical angles' integrals, but nevertheless, just like the pyramid, it occupies one-third of the cylinder into which it can be inscribed.

Therefore, its volume is equal to the product of the base area and the height, divided by three, or the product of π the square of the radius and the height, divided by three.