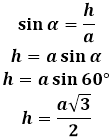Equilateral Triangle Height

An equilateral triangle is a regular polygon (a geometric figure where all angles and all sides are equal). In fact, this significantly simplifies the process of calculating any parameters characterizing such a triangle, including the height.

In an equilateral triangle, all three heights are of equal length, so having found any one of them, you can apply the obtained value to all three lines. Moreover, all the heights coincide completely with all three medians, bisectors and perpendicular bisectors, otherwise known as mediatrises. The point of intersection of all three lines possesses the properties of the point of intersection of the heights, the point of intersection of the medians, and the point of intersection of the bisectors simultaneously, representing any of the possible triangle centers, including the center of the inscribed and circumscribed circles.

Based on this, to find the height of an equilateral triangle, you can use absolutely any known parameters, for example, the side of the triangle.

The height of an equilateral triangle, drawn to any side, creates a right triangle inside it, which can be calculated using trigonometric relationships, as it is known that all angles in an equilateral triangle are 60 degrees. For the obtained right triangle, the height will be a leg, opposite the 60-degree angle, and the side of the equilateral triangle is the hypotenuse, accordingly, to find the height, you need to apply the sine. If you substitute 60 degrees for angle alpha, it turns out that the height of the equilateral triangle is half the side multiplied by the square root of three.