Trapezoid Diagonals
A trapezoid is an unconventional quadrilateral in which two of the sides – the bases of the trapezoid, are parallel to each other. If you draw diagonals in a trapezoid, they form a series of similar triangles, and the proportional relationships of their sides lead to the main property of the trapezoid, combining the diagonals of the trapezoid and its four sides:
d12+d22=c2+d2+2ab , where a and b – these are the bases of the trapezoid, and c and d – its lateral sides.
These same properties of the similar and equal triangles formed by the diagonals determine the following separate formulas for the trapezoid diagonals through the sides:
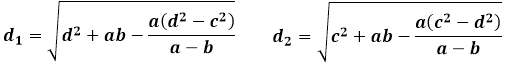
In the given formulas, the length of the diagonal d1 denotes the diagonal of the trapezoid, which forms a triangle with the base of the trapezoid a and the lateral side d, and the length of the diagonal d2 is equal in value, respectively, to the line connecting the base of the trapezoid b and the lateral side c.
