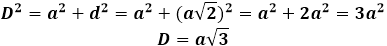Cube Diagonal

A cube is a basic geometric body when it comes to volume and volumetric bodies. Not surprisingly, the third power, which is obtained by multiplying three identical numbers together (as when finding the volume of a cube - three of its identical measurements) is named in its honor.

The main and only parameter of the cube is its edge a, since all edges of a cube are congruent and represent both length, width, and height. Accordingly, only one value determines all possible characteristics of the cube related to its dimensions.

In addition to the edges, the cube's vertices can be connected by diagonals. Diagonals can pass through the cube faces, in which case they will simply be the base diagonal or the square diagonal in the plane, or the diagonals can be drawn inside the cube itself, connecting opposite bases at extreme points (vertices).

To find the cube diagonal through its edge, you first need to make an additional construction in the form of the diagonal of one of the connected bases, then the cube diagonal becomes the hypotenuse of the newly formed right triangle, whose legs are the cube's edge and the base diagonal. If the edge of the cube is given by the conditions of the problem, then the square diagonal at the base must first be calculated using the formula: d=a√2

Then the cube diagonal can be expressed through the Pythagorean theorem, and it will take the following form: